Github团队协作流程上
本文共 513 字,大约阅读时间需要 1 分钟。
GitHub Flow是一个非常轻便的,基于分支的工作流。
非常适合代码部署非常频繁的团队和项目。
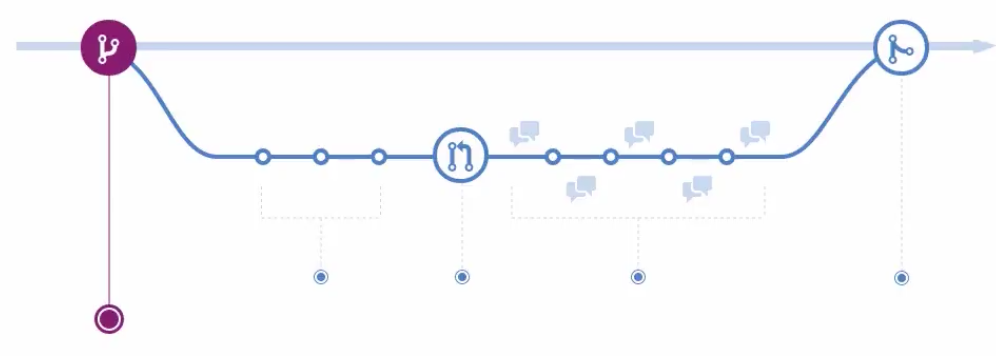
一 工作流步骤
第1步:创建新分支
第2步:在新分支的基础上添加新版本
第3步:开启一个Pull Request
第4步:讨论和代码审核
第5步:合并分支,然后部署
二 工作流方式
1、团队成员之间合作方式
2、开源项目中和互不相识的开源贡献者合作
三 给队友添加写权限
1 场景
cakin24给chengqiuming添加写权限
2 步骤
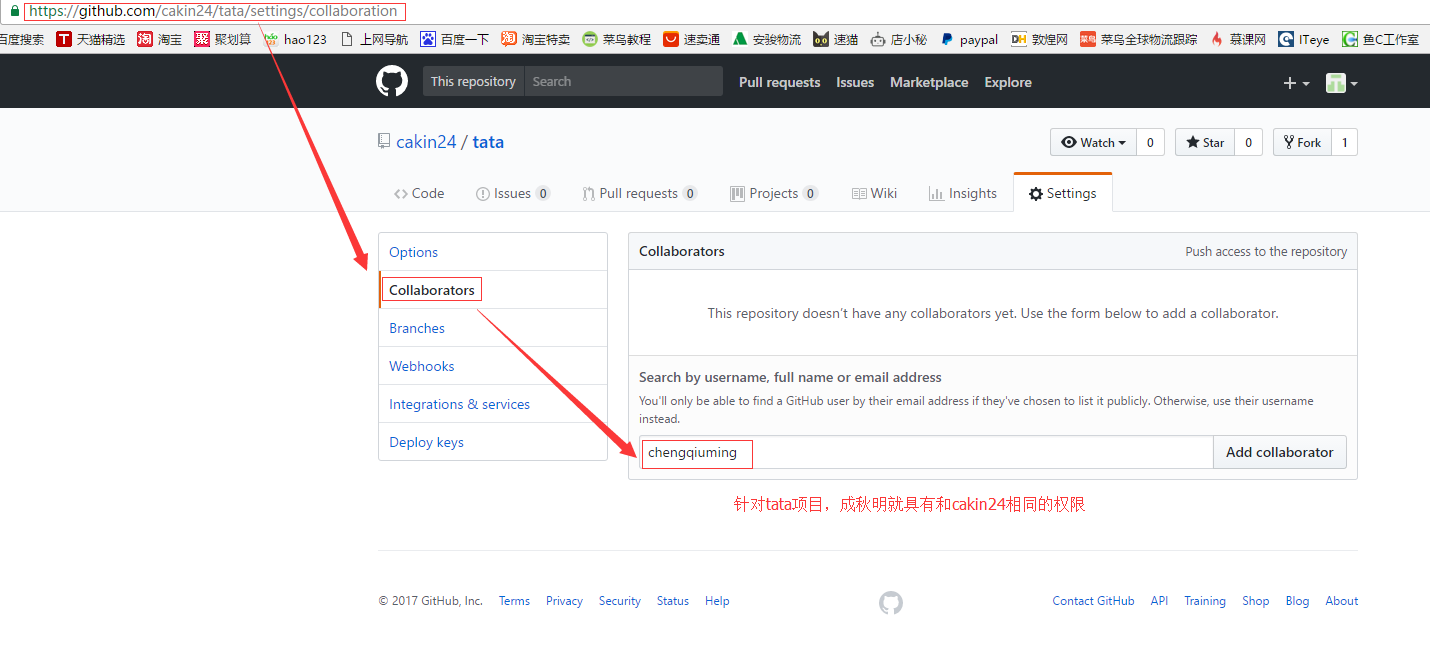
2.1 cakin24进入tata项目的setting页面
2.2 cakin24按照如下方式进行设置,添加chengqiuming的写权限
四 cakin24进行开发流程
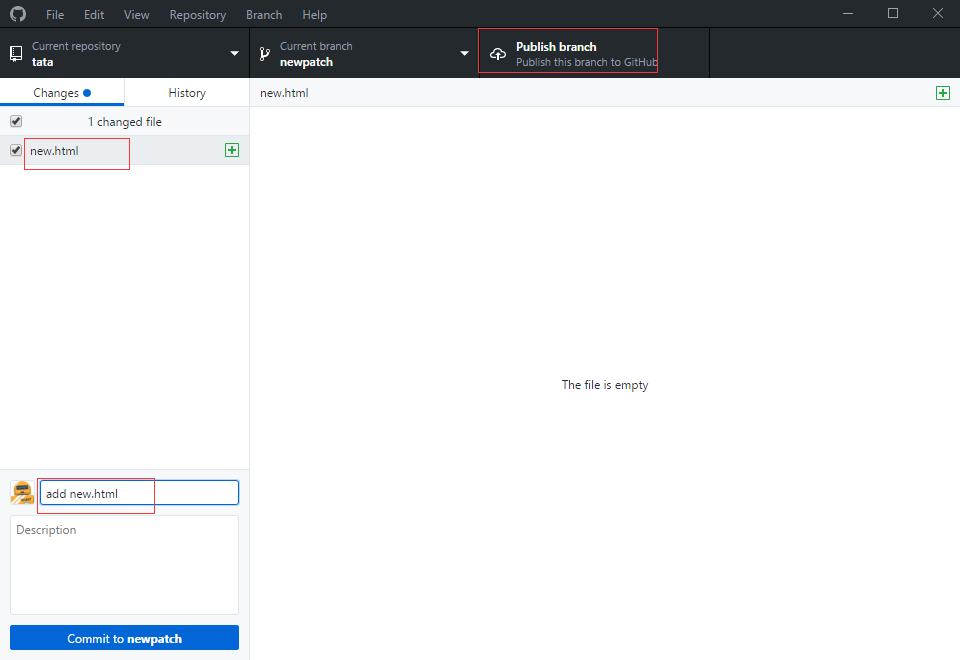
1 cakin24针对tata项目新建立一个分支,并添加文件new.html,然后发布
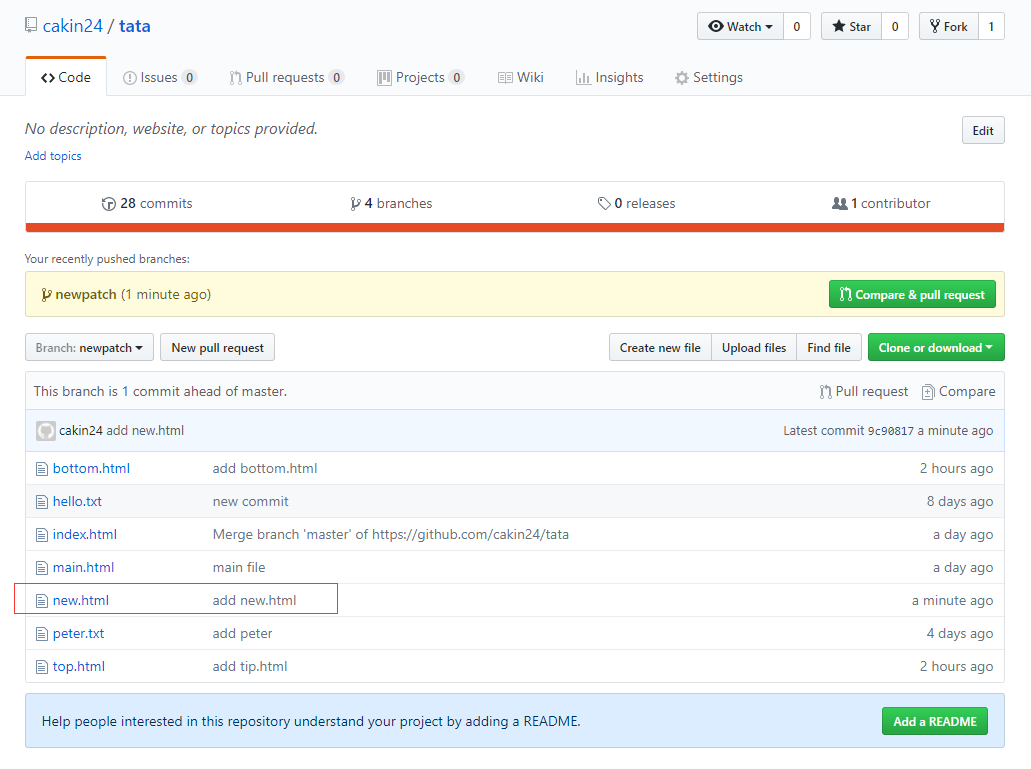
2 cakin24登录github,发现新的分支已创建
3 点击New pull request

4 点击Create pull request
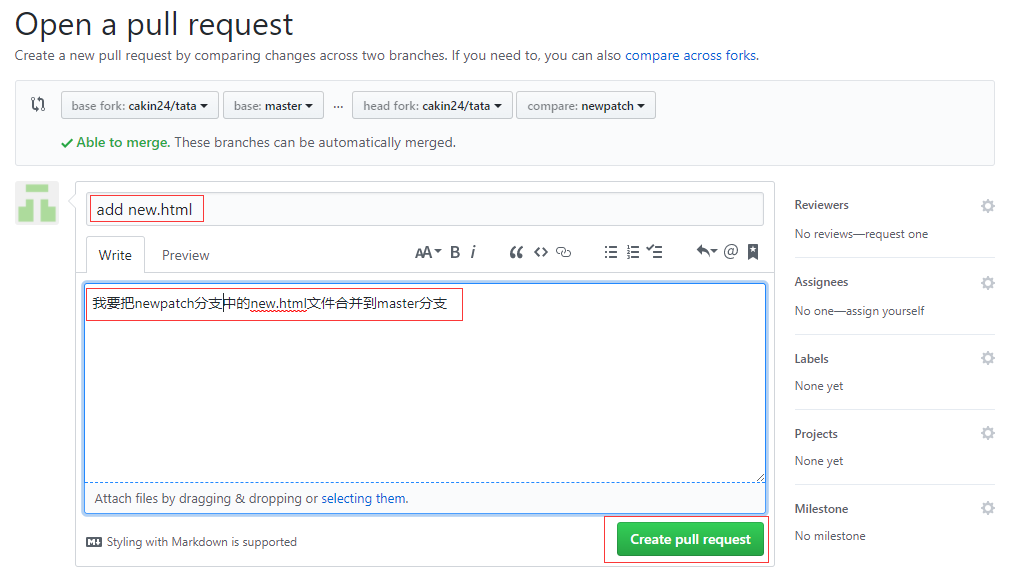
5 点击Merge pull request
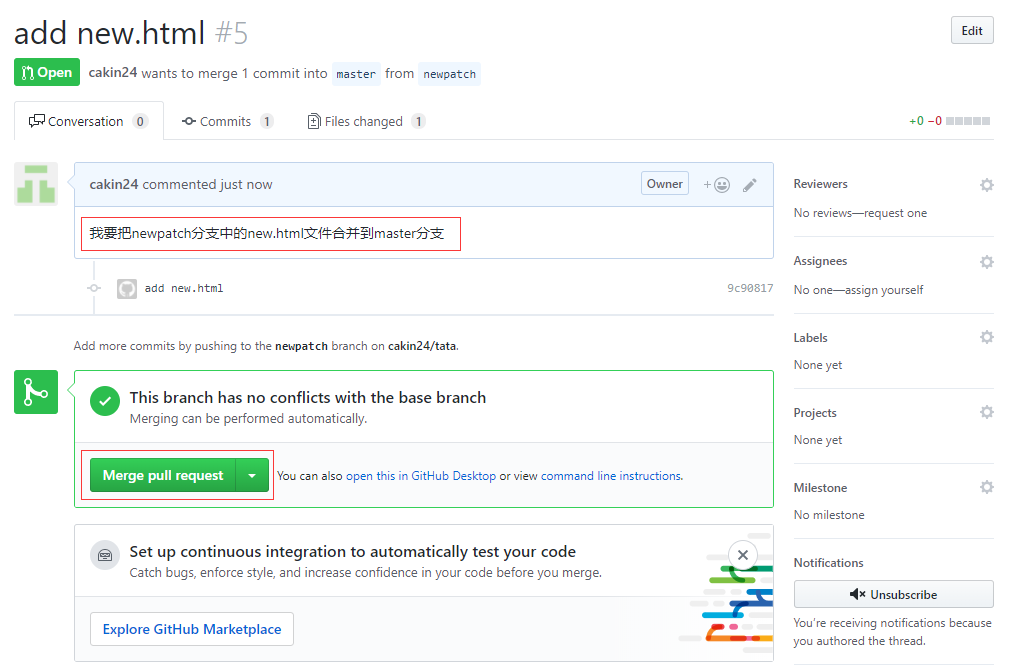
6 点击Confirm request,确认合并
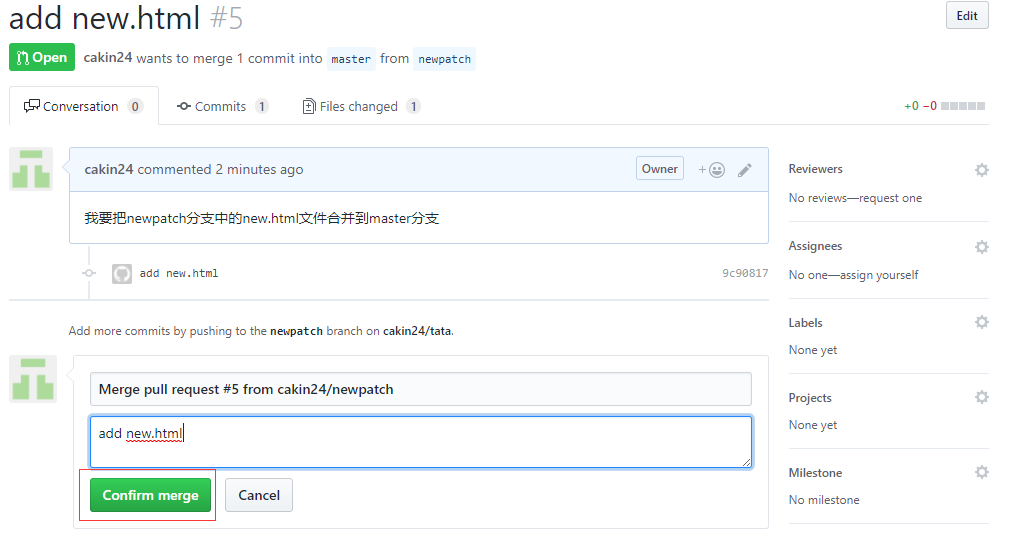
7 点击Comment,发表一下合并成功评论
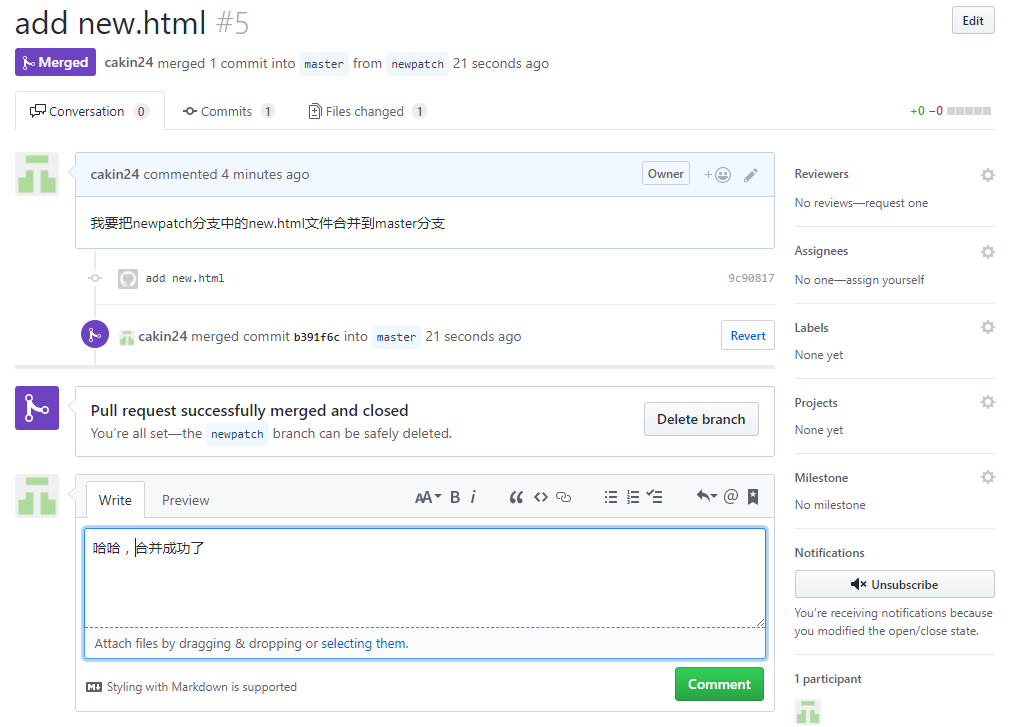
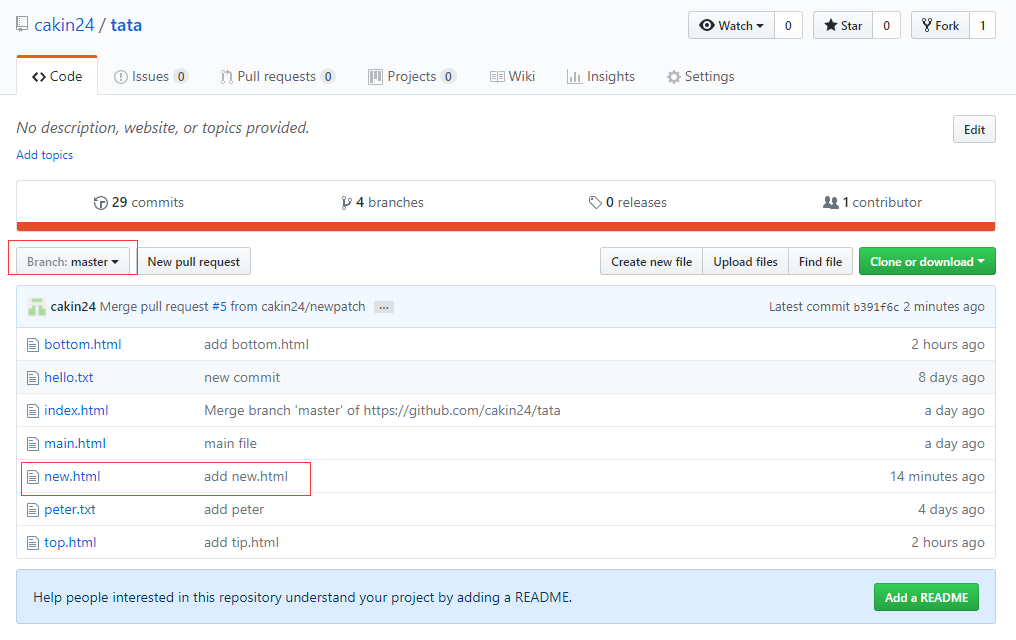
8 发现master项目被合并了
你可能感兴趣的文章
Mysql启动失败解决过程
查看>>
MySQL启动失败:Can't start server: Bind on TCP/IP port
查看>>
mysql启动报错
查看>>
mysql启动报错The server quit without updating PID file几种解决办法
查看>>
MySQL命令行登陆,远程登陆MySQL
查看>>
mysql命令:set sql_log_bin=on/off
查看>>
mySQL和Hive的区别
查看>>
MySQL和Java数据类型对应
查看>>
mysql和oorcale日期区间查询【含左右区间问题】
查看>>
MYSQL和ORACLE的一些操作区别
查看>>
mysql和redis之间互相备份
查看>>
MySQL和SQL入门
查看>>
mysql在centos下用命令批量导入报错_Variable ‘character_set_client‘ can‘t be set to the value of ‘---linux工作笔记042
查看>>
Mysql在Linux运行时新增配置文件提示:World-wrirable config file ‘/etc/mysql/conf.d/my.cnf‘ is ignored 权限过高导致
查看>>
Mysql在Windows上离线安装与配置
查看>>
MySQL在渗透测试中的应用
查看>>
Mysql在离线安装时启动失败:mysql服务无法启动,服务没有报告任何错误
查看>>
Mysql在离线安装时提示:error: Found option without preceding group in config file
查看>>
MySQL基于SSL的主从复制
查看>>
Mysql基本操作
查看>>